Chapter 4

Types of Chemical Reactions and Solution Stoichiometry

Shaun Williams, PhD

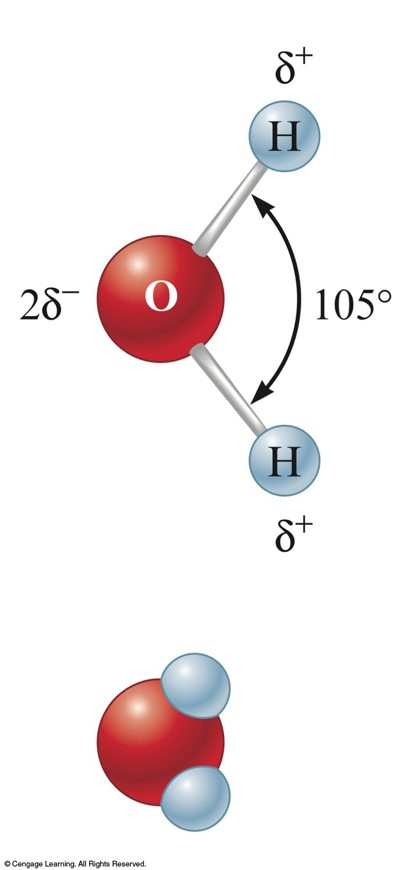
Water, the Common Solvent

Water

• One of the most important substances on Earth.
• Can dissolve many different substances.
• A polar molecule because of its unequal charge distribution.

The Nature of Aqueous Solutions: Strong and Weak Electrolytes

Nature of Aqueous Solutions

• Solute – substance being dissolved.
• Solvent – liquid water.
• Electrolyte – substance that when dissolved in water produces a solution that can conduct electricity.

Electrolytes

• Strong Electrolytes – conduct current very efficiently (bulb shines brightly). Completely ionized in water.
• Weak Electrolytes – conduct only a small current (bulb glows dimly). A small degree of ionization in water.
• Nonelectrolytes – no current flows (bulb remains unlit). Dissolves but does not produce any ions.

The Composition of Solutions

Chemical Reactions of Solutions

• We must know:
  • The nature of the reaction.
  • The amounts of chemicals present in the solutions.

Molarity

• Molarity (M) = moles of solute per volume of solution in liters: $$ \chem{M} = \text{Molarity} = \frac{\text{moles of solute}}{\text{liters of solution}} $$ $$ 3\,\chem{M}\,\chem{HCl} = \frac{6\,\text{moles of HCl}}{2\,\text{liters of solution}} $$

Exercise 1

A 500.0-g sample of potassium phosphate is dissolved in enough water to make 1.50 L of solution. What is the molarity of the solution?

Exercise 1 - Answer

A 500.0-g sample of potassium phosphate is dissolved in enough water to make 1.50 L of solution. What is the molarity of the solution?

Concentration of Ions

• For a \(0.25\,\chem{M}\,\chem{CaCl_2}\) solution: $$ \chem{CaCl_2 \rightarrow Ca^{2+} + 2Cl^-} $$
  • \(\chem{Ca^{2+}}:\,1\times 0.25\,\chem{M} = 0.25\,\chem{M}\,\chem{Ca^{2+}}\)
  • \(\chem{Cl^{-}}:\,2\times 0.25\,\chem{M} = 0.50\,\chem{M}\,\chem{Cl^{-}}\)

Notice

• The solution with the greatest number of ions is not necessarily the one in which:
  • the volume of the solution is the largest.
  • the formula unit has the greatest number of ions.

Dilution

• The process of adding water to a concentrated or stock solution to achieve the molarity desired for a particular solution.
• Dilution with water does not alter the numbers of moles of solute present.
• Moles of solute before dilution = moles of solute after dilution $$ M_1V_1 = M_2V_2 $$

Types of Chemical Reactions

• Precipitation Reactions
• Acid–Base Reactions
• Oxidation–Reduction Reactions

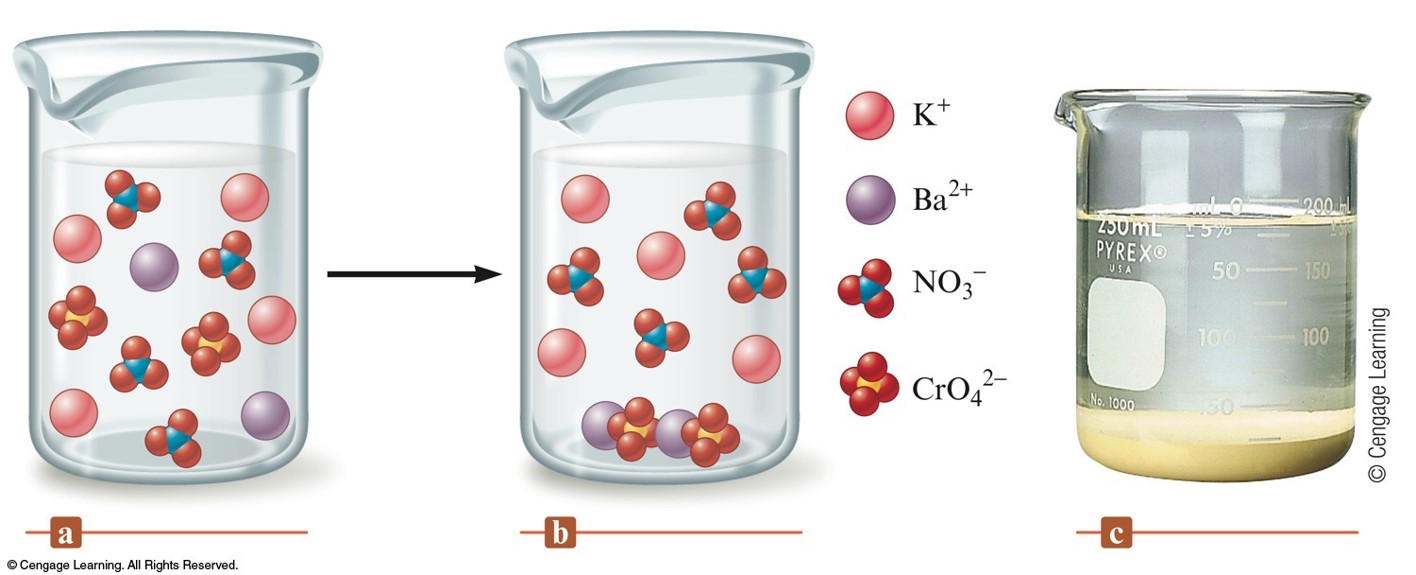
Precipitation Reactions

Precipitation Reaction

• A double displacement reaction in which a solid forms and separates from the solution.
  • When ionic compounds dissolve in water, the resulting solution contains the separated ions.
  • Precipitate – the solid that forms.

\( \chem{Ba\left(NO_3\right)_2(aq)+K_2CrO_4(aq)\rightarrow 2KNO_3(aq) + BaCrO_4(s)} \)

Precipitates

• Soluble – solid dissolves in solution; (aq) is used in reaction equation.
• Insoluble – solid does not dissolve in solution; (s) is used in reaction equation.
• Insoluble and slightly soluble are often used interchangeably.

Simple Rules for Solubility

1. Most nitrate (\(\chem{NO_3^-}\)) salts are soluble.
2. Most alkali metal (group 1A) salts and \(\chem{NH_4^+}\) are soluble.
3. Most \(\chem{Cl^-}\), \(\chem{Br^-}\), and \(\chem{I^-}\) salts are soluble (except \(\chem{Ag^+}\), \(\chem{Pb^{2+}}\), \(\chem{Hg_2^{2+}}\)).
4. Most sulfate salts are soluble (except \(\chem{BaSO_4}\), \(\chem{PbSO_4}\), \(\chem{Hg_2SO_4}\), \(\chem{CaSO_4}\)).
5. Most \(\chem{OH^-}\) are only slightly soluble (\(\chem{NaOH}\), \(\chem{KOH}\) are soluble, \(\chem{Ba(OH)_2}\), \(\chem{Ca(OH)_2}\) are marginally soluble).
6. Most \(\chem{S^{2-}}\), \(\chem{CO_3^{2-}}\), \(\chem{CrO_4^{2-}}\), \(\chem{PO_4^{3-}}\) salts are only slightly soluble, except for those containing the cations in Rule 2.

Describing Reactions in Solution

Formula Equation (Molecular Equation)

• Gives the overall reaction stoichiometry but not necessarily the actual forms of the reactants and products in solution.
• Reactants and products generally shown as compounds.
• Use solubility rules to determine which compounds are aqueous and which compounds are solids.

$$ \chem{AgNO_3(aq)+NaCl(aq)\rightarrow AgCl(s)+NaNO_3(aq)} $$

Complete Ionic Equation

• All substances that are strong electrolytes are represented as ions.

$$ \begin{align} \chem{Ag^+(aq)+NO_3^-(aq)+} & \chem{Na^+(aq)+Cl^-(aq)\rightarrow} \\ & \chem{AgCl(s)+Na^+(aq)+NO_3^-(aq)} \end{align} $$

• Includes only those solution components undergoing a change.
  • Show only components that actually react.
  $$ \chem{Ag^+(aq)+Cl^-(aq)\rightarrow AgCl(s)} $$
• Spectator ions are not included (ions that do not participate directly in the reaction).
  • \(\chem{Na^+}\) and \(\chem{NO_3^-}\) are spectator ions.

Stoichiometry of Precipitation Reactions

Solving Stoichiometry Problems for Reactions in Solution

1. Identify the species present in the combined solution, and determine what reaction occurs.
2. Write the balanced net ionic equation for the reaction.
3. Calculate the moles of reactants.
4. Determine which reactant is limiting.
5. Calculate the moles of product(s), as required.
6. Convert to grams or other units, as required.

Concept Check 1

10.0 mL of a 0.30 M sodium phosphate solution reacts with 20.0 mL of a 0.20 M lead(II) nitrate solution (assume no volume change).

• What precipitate will form $$ \phantom{ } $$
• What mass of precipitate will form? $$ \phantom{ } $$

Concept Check 1 - Answer

10.0 mL of a 0.30 M sodium phosphate solution reacts with 20.0 mL of a 0.20 M lead(II) nitrate solution (assume no volume change).

• What precipitate will form $$ \text{lead(II) phosphate, } \chem{Pb_3\left(PO_4\right)_2} $$
• What mass of precipitate will form? $$ 1.1\,\chem{g}\,\chem{Pb_3\left(PO_4\right)_2} $$

Acid-Base Reactions

Acid-Base Reactions (Brønsted-Lowry)

• Acid—proton donor
• Base—proton acceptor
• For a strong acid and base reaction: $$ \chem{H^+(aq)+OH^-(aq)\rightarrow H_2O(l)} $$

Performing Calculations for Acid-Base Reactions

1. List the species present in the combined solution before any reaction occurs, and decide what reaction will occur.
2. Write the balanced net ionic equation for this reaction.
3. Calculate moles of reactants.
4. Determine the limiting reactant, where appropriate.
5. Calculate the moles of the required reactant or product.
6. Convert to grams or volume (of solution), as required.

Acid-Base Titrations

• Titration – delivery of a measured volume of a solution of known concentration (the titrant) into a solution containing the substance being analyzed (the analyte).
• Equivalence point – enough titrant added to react exactly with the analyte.
• Endpoint – the indicator changes color so you can tell the equivalence point has been reached.

Concept Check 2

For the titration of sulfuric acid (\(\chem{H_2SO_4}\)) with sodium hydroxide (\(\chem{NaOH}\)), how many moles of sodium hydroxide would be required to react with 1.00 L of 0.500 M sulfuric acid to reach the endpoint? $$ \phantom{ } $$

Concept Check 2 - Answer

For the titration of sulfuric acid (\(\chem{H_2SO_4}\)) with sodium hydroxide (\(\chem{NaOH}\)), how many moles of sodium hydroxide would be required to react with 1.00 L of 0.500 M sulfuric acid to reach the endpoint? $$ 1.00\,\chem{mol}\,\chem{NaOH} $$

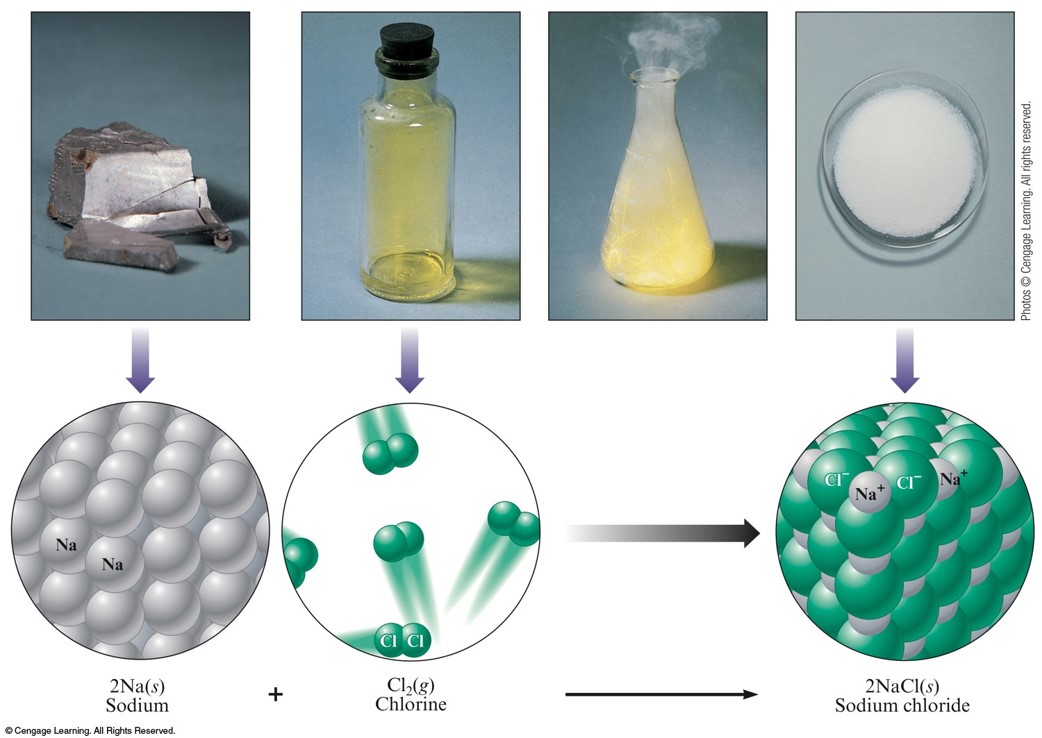
Oxidation-Reduction Reactions

Redox Reactions

• Reactions in which one or more electrons are transferred.

Reaction of Sodium and Chlorine

Rule for Assigning Oxidation States

1. Oxidation state of an atom in an element = 0
2. Oxidation state of monatomic ion = charge of the ion
3. Oxygen = -2 in covalent compounds (except in peroxides where it = -1)
4. Hydrogen = +1 in covalent compounds
5. Fluorine = -1 in compounds
6. Sum of oxidation states = 0 in compounds
7. Sum of oxidation states = charge of the ion in ions

Exercise 1

Find the oxidation states for each of the elements in each of the following compounds:

• \(\chem{K_2Cr_2O_7}\)
• \(\chem{CO_3^{2-}}\)
• \(\chem{MnO_2}\)
• \(\chem{PCl_5}\)
• \(\chem{SF_4}\)

Exercise 1 - Answers

Find the oxidation states for each of the elements in each of the following compounds:

• \(\chem{K_2Cr_2O_7}\) \(\Rightarrow \chem{K=+1;\, Cr=+6;\, O=-2}\)
• \(\chem{CO_3^{2-}}\) \(\Rightarrow \chem{C=+4;\, O=-2}\)
• \(\chem{MnO_2}\) \(\Rightarrow \chem{Mn=+4;\, O=-2}\)
• \(\chem{PCl_5}\) \(\Rightarrow \chem{P=+5;\, Cl=-1}\)
• \(\chem{SF_4}\) \(\Rightarrow \chem{S=+4;\, F=-1}\)

Redox Characteristics

• Transfer of electrons
• Transfer may occur to form ions
• Oxidation – increase in oxidation state (loss of electrons); reducing agent
• Reduction – decrease in oxidation state (gain of electrons); oxidizing agent

Balancing Oxidation-Reduction Equations

Balancing Oxidation-Reduction Reactions by Oxidation States

1. Write the unbalanced equation.
2. Determine the oxidation states of all atoms in the reactants and products.
3. Show electrons gained and lost using "tie lines."
4. Use coefficients to equalize the electrons gained and lost.
5. Balance the rest of the equation by inspection.
6. Add appropriate states.

Example 1

Balance the reaction between solid zinc and aqueous hydrochloric acid to produce aqueous zinc(II) chloride and hydrogen gas.

1. What is the unbalanced equation? $$ \chem{Zn(s)+HCl(aq)\rightarrow Zn^{2+}(aq)+Cl^-(aq)+H_2(g)} $$
2. What are the oxidation states for each atom? $$ \chem{\underbrace{Zn}_0(s)+\underbrace{H}_{+1}\underbrace{Cl}_{-1}(aq)\rightarrow \underbrace{Zn^{2+}}_{+2}(aq)+\underbrace{Cl^-}_{-1}(aq)+\underbrace{H_2}_0(g)} $$
3. How are electrons gained and lost? $$ \rlap{\overbrace{\phantom{\chem{Zn(s)+HCl(aq)\rightarrow Zn}}}^\text{1 electron gained (each atom)}}\chem{\underbrace{Zn}_0(s)}+\underbrace{\chem{\underbrace{H}_{+1}\underbrace{Cl}_{-1}(aq)}\rightarrow \chem{\underbrace{Zn^{2+}}_{+2}(aq)}+\chem{\underbrace{Cl^-}_{-1}(aq)}+\chem{\underbrace{H_2}_0}}_\text{2 electrons lost} \chem{(g)} $$ The oxidation state of chlorine remains unchanged.

Example 1 - cont.

4. What coefficients are needed to equalize the electrons gained and lost? $$ \rlap{\overbrace{\phantom{\chem{Zn(s)+HCl(aq)\rightarrow Zn}}}^\text{1 electron gained (each atom) $\times$ 2}}\chem{\underbrace{Zn}_0(s)}+\underbrace{\chem{\underbrace{H}_{+1}\underbrace{Cl}_{-1}(aq)}\rightarrow \chem{\underbrace{Zn^{2+}}_{+2}(aq)}+\chem{\underbrace{Cl^-}_{-1}(aq)}+\chem{\underbrace{H_2}_0}}_\text{2 electrons lost} \chem{(g)} $$ $$ \chem{Zn(s) + 2HCl(aq) \rightarrow Zn^{2+}(aq)+Cl^-(aq)+H_2(g)} $$
5. What coefficients are needed to balance the remaining elements? $$ \chem{Zn(s) + 2HCl(aq) \rightarrow Zn^{2+}(aq)+2Cl^-(aq)+H_2(g)} $$